The two common symmetric chords are the augmented triad, dividing the octave in major thirds, and the diminished 7th chord, dividing the octave in minor thirds:

When we transpose non-symmetric chords, we all know that going up an interval lead to the same chord as going down the inverted interval. For instance, if I transpose a C chord up a perfect fourth, i got a F chord … as well as if I transpose my C chord down a perfect fifth (inversion of the perfect fourth). Obvious:
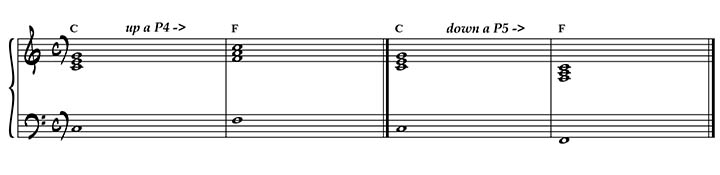
Although this also apply to symmetric chords, these ones got other interesting properties regarding transposition. Because they divide the octave in equal parts, they have a limited number of transpositions. We know that Caug contains the same notes as Eaug and Abaug … so they can be completely interchanged in music. Same thing for Cdim7, Ebdim7, F#dim7 and Adim7. They are enharmonic chords:

Now let’s combine the two previous concepts. What happens if I transpose a Caug chord down a perfect fifth ? I got a Faug, right ? But Faug is also enharmonically a Aaug and a Dbaug. This means that for augmented chords, going down a perfect fifth (or up a perfect fourth) is an equivalent harmonic movement as going down a minor third (or up a major sixth) and as going up a minor second (or down a major seventh):
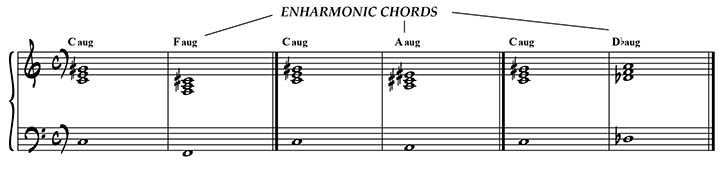
Same idea applied to dim7 chords. When I transpose a Cdim7 down a perfect fifth, i got a Fdim7 which is also enharmonically a Abdim7, Bdim7 and Ddim7. This means that for dim7 chords, going down a perfect fifth (or up a perfect fourth) is an equivalent harmonic movement as going down a major third (or up a minor third), down a minor second (or up a major seventh) and up a major second (or down a minor seventh):

We could apply the same concept to other transpositions than a perfect fifth down, of course, but this one is particularly interesting because it is the most common harmonic movement in music, as it goes toward the circle of fifths, counter-clockwise. It’s the harmonic movement of the II-V-I and III-VI-II-V progressions, and of numbers of harmonic marches.
Now let’s make some music with this idea ! I picked up a famous jazz standard, Polkadots & Moonbeams, in the key of F. The end of the first A section goes like this:

The last bar of the section is the interesting one. We have here Am7 D7 Gm7 C7 … a typical progression to go back to the F tonic. We can reharmonize it with 7th chords, a very common practice in jazz:
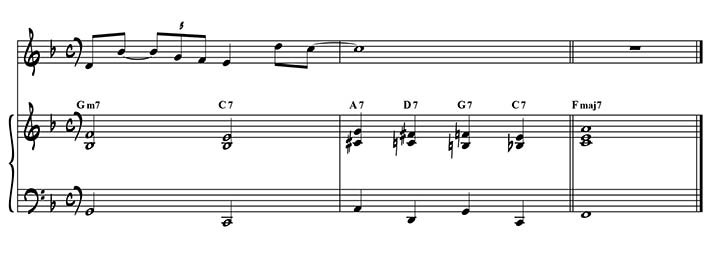
Now we can first substitute these 7th chords by augmented triads, which work very well as a dominant function … A7 D7 G7 C7 becoming Aaug Daug Gaug Caug. But if we apply the concept we just discussed above, instead of going down perfect fifths, we can go up minor seconds and we will still have the same chords, enharmonically: Aaug, Bbaug, Baug, Caug. We can double check that Bbaug is the same chord as Daug, and Baug the same chord as Gaug.

We can also use these ascending voicings with the original bass line, and we go back to the original augmented triads progression with a smooth ascending voice-leading:

Got it ?
Let’s now use dim7 chords. We now that dominant 7th chords can be substitute by dim7 chords … C7 can be substituted by Edim7. This makes sound the Db note, which is the b9 of C7. Also very common practice in jazz. A7 D7 G7 C7 then become C#dim7 F#dim7 Bdim7 Edim7. Again, applying the same concept, instead of going down perfect fifths, we can go down minor seconds, which is an equivalent harmonic movement for dim7 chords. This gives us:

And again, using these dim7 voicings with the original bass line, we’re back to 7(b9) chords. With a drop2 figuration, we get this smooth descending voice-leading:

That’s it for today !
If this website is helping you becoming a better musician, please consider making a donation. Even a small amount will be very appreciated. Thank you !



Leave A Comment